 Z-transform
Z-transform
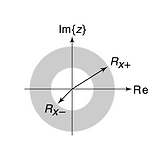
Fourier Transform에는 두 가지 단점이 있다. DTFT가 존재하지 않는 여러 유용한 signal들이 존재한다. 예를 들어, $u(n)$, $nu(n)$과 같은 신호들은 absolutely summable하지 않아서 DTFT가 존재하지 않는다. 또한, 초기 조건이나 input의 변화로 인한 system의 transient response(변화 대응해 steady-state로 가기전 과도기적 상태)를 DTFT에서는 반영하지 못한다. 이러한 두 가지 문제를 해결하기 위해 DTFT의 확장 형태인 z-transform이 나오게 되었다. Bilateral z-transform Definition $$ X(z)\equiv \sum_{n=-\infty}^{\infty} x(n)z^{-n}, R_{-} < ..
digital signal process
2022. 1. 11. 20:15
최근에 올라온 글
TAG
- AI
- ML
- Generative Model
- Depth estimation
- Deep learning
- TRACKING
- feature
- 딥러닝
- 운영체제
- Raspberry Pi
- depthmap
- Gan
- pcb
- Building Basic GAN
- Operating System
- image
- depth
- MLOps
- deeplearning
- ML Pipeline
- DSP
- 3d object detection
- 신호처리
- mode collapse
- OS
- design pattern
- 디지털신호처리
- machine learning
- controllable GAN
- conditional GAN
- Total
- Today
- Yesterday
최근에 달린 댓글
링크
공지사항
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | ||||||
| 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| 9 | 10 | 11 | 12 | 13 | 14 | 15 |
| 16 | 17 | 18 | 19 | 20 | 21 | 22 |
| 23 | 24 | 25 | 26 | 27 | 28 |
글 보관함
